Challenge questions from FPM quizzes: First quiz on sets and subsets

-

 My fourth FPM quiz last autumn was on sets and subsets. Again the "challenge" question isn't too hard, as long as you understand the basic concepts and definitions, though it is easy to make mistakes.

Here is a screenshot of the question. As usual, the buttons don't do anything, but you can enlarge the image by clicking on it.

Quiz question

Click on the screenshot to enlarge the image

Here \(\mathbb{R}\), \(\mathbb{Q}\) and \(\mathbb{Z}\) have their usual meanings, and \(\emptyset\) is the empty set.

You also need to know about the operations of intersection (denoted by \(\cap\)) and set difference (denoted by a backslash, \(\setminus\)) and the "subset" relation (denoted by \(\subseteq\)). Here I use the "subset or equals" notation \(\subseteq\) to make it clear that sets which are equal do count as subsets of each other.

In particular, note that the notation \(Y \nsubseteq X\) means that \(Y\) is not a subset of \(X\), which is equivalent to saying that there is at least one element of \(Y\) that is not an element of \(X\).

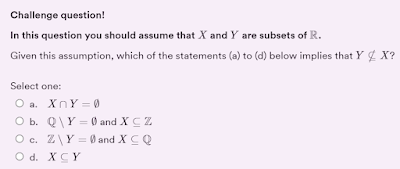
Comments

Post a Comment

Popular posts from this blog

Sums and integration against counting measure: Part I

-
For other posts in this series, see https://explaining-maths.blogspot.com/search?q=Sums+and+integration+against+counting+measure In case the MathJax mathematics below does not display properly in your browser, I have made a PDF of the main article available via my  WordPress blog  at  https://explainingmaths.files.wordpress.com/2023/08/sums-and-integration-against-counting-measure_-part-i.pdf Note added 30/8/23: I'm not quite sure when I started to say "integration against a measure". I'm not alone, but I think that "integration with respect to a measure" is a lot more common! However, I don't want to change the titles of my latest 10 blog posts, so I'll leave it as it is. I'll probably change back to "integration   with respect to a measure" in future though! It has been a while since I wrote a mathematical post! Here I thought I would say something about Measure and Integration which is relevant to my research. For these posts I as
Read more

Discussion of the proof that the uniform norm really is a norm

-
In response to a request on Piazza, I gave a detailed proof that the uniform really is a norm, including some comments and warnings on possible pitfalls along the way. Here (essentially) is what I said. Let’s have a look at \(\|\cdot\|_\infty\) on the bounded, real-valued functions on \([0,1]\) (but you can also work with bounded functions on any non-empty set you like, and the same proof will work). We define \(\ell_\infty([0,1]) = \{f: f \textrm{ is a bounded, real-valued function on }[0,1]\,\}\,,\) and this is the set/space we will look at. So we set \(Y=\ell_\infty([0,1])\). Then you can check for yourselves that \(Y\) really is a vector space over \(\mathbb{R}\) (using the usual "pointwise" operations for functions). Note that the zero element in this vector space is the constant function \(0\) (from \([0,1]\) to \(\mathbb{R}\)), which I'll denote by \(\mathbf{0}\) here for clarity. (We also used \(Z\) for this function in one of the exercises.) I'll first give
Read more

Revisiting liminf and limsup

-
This post is also available in PDF form from my WordPress Blog at  https://explainingmaths.wordpress.com/2023/09/01/revisiting-liminf-and-limsup/ My recent series of posts about Fatou's Lemma for sums was aimed primarily at mathematics students at 3rd-year undergraduate level or above. So I think I should write a post more suitable for first-year undergraduate students. So I'm going to have another look at the topic of $\liminf$ and $\limsup$ for bounded sequences of real numbers (though most of what I say can be generalised to sequences, or even nets, of extended real numbers). I said quite a lot about this in a previous post on my WordPress blog in the post https://explainingmaths.wordpress.com/2009/01/10/an-application-of-absorption-to-teaching-lim-inf-and-lim-sup/ and there was a lot of discussion in the comments below the post. In particular, in those comments, I discussed the $\liminf$ and the $\limsup$ of some examples of sequences $(x_n)$, including the following exam
Read more